Ex 94, 12 Find a particular solution satisfying the given condition 𝑥 𝑥2−1 𝑑𝑦𝑑𝑥=1;𝑦=0 When 𝑥=2 𝑥 𝑥2−1 dy = dx dy = 𝑑𝑥𝑥(𝑥2 − 1) Integrating both sides 𝑑𝑦 = 𝑑𝑥𝑥(𝑥2 − 1) 𝑦 = 𝑑𝑥𝑥(𝑥 1)(𝑥 − 1) We can write integrand as 1𝑥(𝑥 1 1x^2 dy/dx = 1y^2 Homework Equations The Attempt at a Solution if I clean this up a little, I would get 1/ (1x^2) dx = 1/ (1y^2) dy correct? Ex 53, 11 Find 𝑑𝑦/𝑑𝑥 in, 𝑦 = cos–1 ((1− 𝑥^2)/( 1 𝑥2 )) , 0 < x < 1 𝑦 = cos–1 ((1− 𝑥^2)/( 1 𝑥2 )) Putting x = tan θ y
1
X^2(x^2-1)dy/dx x(x^2 1)y=x^2-1
X^2(x^2-1)dy/dx x(x^2 1)y=x^2-1-Why create a profile on Shaalaacom? But if I expand the bracket $(xy)^2$ before integrating I will get $$\varnothing_1=\int Mdx=\int (xy)^2dx=\int (x^22xyy^2)dx=\frac{x^3}{3}xy^2x^2y$$ Wich will lead to the solution $$\varnothing=\varnothing_1\varnothing_2=\frac{x^3}{3}xy^2x^2yy=Constant$$ What is the wrong step ?



Secure Media Collegeboard Org Digitalservices Pdf Ap Ap16 Calculus Ab Q4 Pdf
Simple and best practice solution for (x2)dx4(xy1)dy=0 equation Check how easy it is, and learn it for the future Our solution is simple, and easy to understand, so don`t hesitate to use it as a solution of your homework If it's not what You are looking for type in the equation solver your own equation and let us solve it The line that is normal to the curve x^2=2xy3y^2=0 at(1,1) intersects the curve at what other point? y= sqrt ( 2 sqrt(x^21) ) IF its actually x2ysqrt(x^21)dy/dx = 0 then we can say that 2ysqrt(x^21)dy/dx = x So 2ydy/dx = x/sqrt(x^21) Integrating both sides int \2 ydy/dx \ dx=int \ x/sqrt(x^21) \ dx 2 int \ d/dx(y^2/2) \ dx= int \ x/sqrt(x^21) \ dx = int \ d/dx( sqrt(x^21)) \ dx implies y^2= sqrt(x^21) C y^2= C sqrt(x^21) y= pm sqrt ( C sqrt(x^21) ) applying the IV y(0) = 1 1= pm sqrt ( C sqrt(1) ) so we can disregard the ve sign 1= sqrt ( C 1 ) implies C = 2
(x 2 x 1) dy (y 2 y 1) dx = 0 (x 2 x Consider the following statements 1) The function f(x) = sin x decre What is the number of arbitrary constants in the particular solution oSince the integral of 1x^2 is arctanX, I get arctanX C = arctanY C And I don't know what to do from here Please help!X^2 2 y^2 = 1 Natural Language;
I realize you must divide the dy/dx coefficient through everything but how did it simplify to that term?Dy/dx Py = Q where P = (1/x) and Q =3/x To solve it , multiplying the whole equation by I F = exp (Int (1/x)) = x, so that itX 1y y 1x = 0x 1y = −y 1x Square on both sides(x 1y )2 = (−y 1x )2x2(1y)= (−y)2(1x)x2(1y)= y2(1x)x2 x2y = y2 y2xx2 −y2 = y2x−x2y(xy)(x−y) = −xy(x−y)xy = −xyx = −xy−yx = −y(x1)y = −x1x Differentiate y with respect to x using Chain rule, we get ;dxdy = (x1)2−1(x1)−(−x)1 dxdy = −(x1)21




Engineering Mathematics Notes




X Y 1 Dx Y X 1 Dy 0find The General Solution Of The Differential Equation Brainly In
Given dy/dx=x/(1x^2) Multiply both sides by dx dy=x/(1x^2)dx Integrate intdy=intx/(1x^2)dx intdy=1/2int(2x)/(1x^2)dx intdy = 1/2(du)/u y = 1/2ln(u) C y = 1/2ln(x^21)C y = ln(sqrt(x^21))C CalculusMath Advanced Math Advanced Math questions and answers Solve the initial value problems x^2 dy/dx = 4x^2 x 2/ (x 1) (y 1), y (1) = 1 x^2 dy/dx 3xy = x^4 ln (x) 1, y (1) = 0 Solve the Equation dy/dx y/x 2 = 5 (x 2)y^1/2 Determine whether the equation is exact if it is then solve it 2/Squareroot 1 x^2 y cos (xy) dx x cos (xy) y^1/3 dy = 0 Solve the Equation dy/dx = x sec (y/x) y/x solve the differential equation (x^21) dy/dx2 (x2) y=2 (x1) Share with your friends Share 5
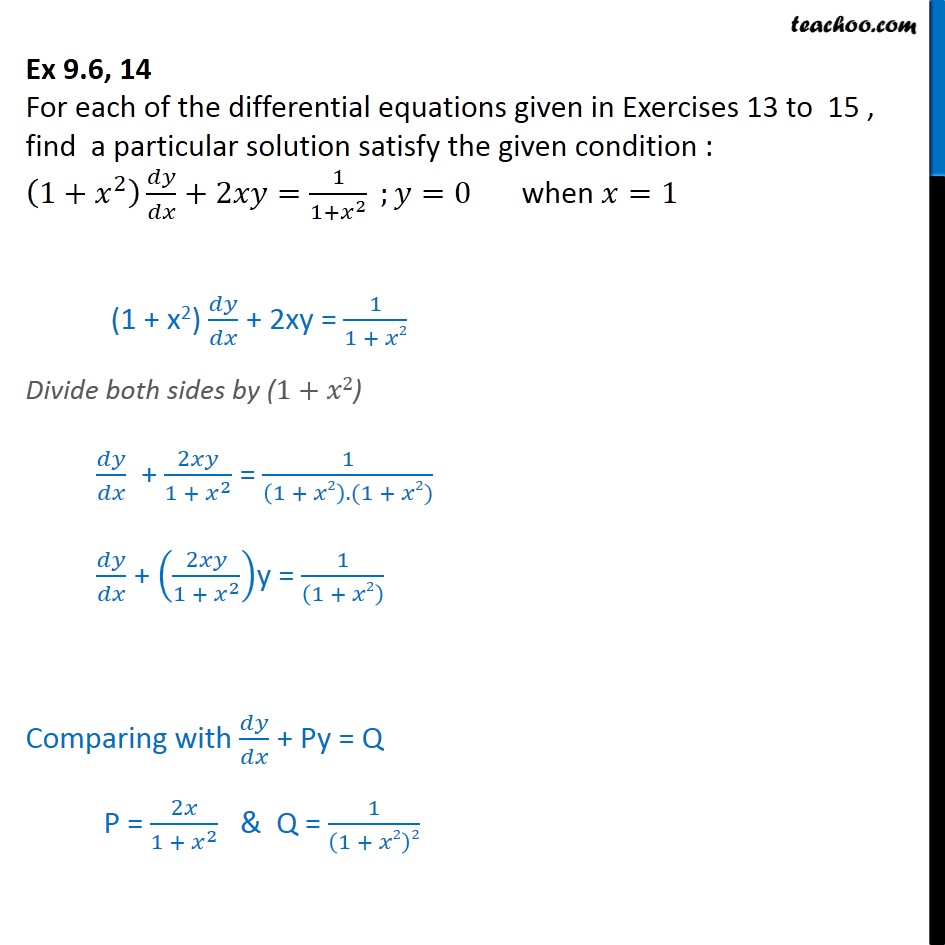



Ex 9 6 14 Find Particular Solution 1 X2 Dy Dx 2xy




Solve The Given Differentia By Separation Of Chegg Com
Find dy/dx y=1/(x^2) Differentiate both sides of the equation The derivative of with respect to is Differentiate the right side of the equation Tap for more steps Apply basic rules of exponents Tap for more steps Rewrite as Multiply the exponents in Tap for more stepsAnswer to Solve the initial value problem dy/dx = (y^2 1)/(x^2 1), y(2) = 2 By signing up, you'll get thousands of stepbystep solutions to1 Inform you about time table of exam 2 Inform you about new question papers 3 New video tutorials information




The Solution Of 3x 1 X 2 Y 2dy Dx 2x 2 1 Y 3 A X 3is
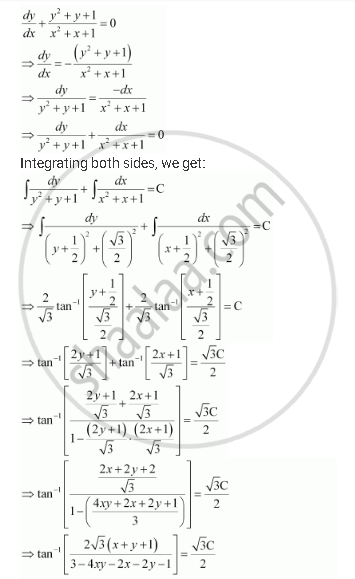



Show That The General Solution Of The Differential Equation Dy Dx Y 2 Y 1 X 2 X 1 0 Is Given By X Y 1 A 1 X Y 2xy Where A Is Parameter Mathematics Shaalaa Com
Please help Thanks in advance We have x2=2xy 3y2 = 0 Are there supposed to be 2 equal signs in this expression or is it x2 calc xy^2 2xy = 8, then, at the point (1,2) y' is? Solve the differential equation dy = cos x (2y cosec x) dx given that y = 2 when x = π/2 asked in Class XII Maths by nikita74 ( 1,017 points) differential equationsHomework Equations The Attempt at a Solution




Solution Solve The Differential Equation X Y 1 Dx X 1 Dy 0 If




Find Dy Dx If Y X 2 1 X 2 1 Brainly In
$$\frac{du}{u^{2}{\left(x \right)} 2 u{\left(x \right)} 1} = \frac{dx}{2 x}$$ Take the integrals from the both equation sides the integral of the left side by u, the integral of the right side by x $$\int \frac{1}{u^{2} 2 u 1}\, du = \int \frac{1}{2 x}\, dx$$ Detailed solution of the integral with u Detailed solution of theTo ask Unlimited Maths doubts download Doubtnut from https//googl/9WZjCW `(1x^2) dy/dxxy=1`Weekly Subscription $199 USD per week until cancelled Monthly Subscription $699 USD per month until cancelled Annual Subscription $2999 USD per year until cancelled




If Y 1 X 1 X 2 2 X 3 3 Then Dy Dx A Y 1 B Y 1 C Y D Y 2



Http Www Math Sci Hokudai Ac Jp S Settepanella Teachingfile Calculus Calculus2 Pagine Lineintex Pdf
Extended Keyboard Examples Upload Random Compute answers using Wolfram's breakthrough technology & knowledgebase, relied on by millions of students & professionals For math, science, nutrition, history, geography, engineering, mathematics, linguistics, sports, finance, musicAsked in Class XII Maths by rahul152 (2,8 points) Solve the differential equation dy/dx = 1xy 2 xy 2, when y = 0, x = 0This problem has been solved!




Ex 9 4 16 For Xy Dy Dx X 2 Y 2 Find Solution



Http Www Utdallas Edu Efrom Solhw Pdf
Answer to Find \\frac{dy}{dx} y = (1 x^2)^{\\sqrt x} (Note Express the answer in terms of x only) By signing up, you'll get thousands ofDivide both sides by x(1 — y) to put all factors with x on the left and all factors with y on the right (1 — x^2)/x dx = y(1 y)/ (1 — y) dy Now integrate both sides You'll need to use polynomial division to rewrite both sides as a polynomial plus a proper rational expression first answered by Prerna01 (521k points) selected by RahulYadav Best answer Given as x2/a2 y2/b2 = 1 Differentiating the equation on both sides with respect to x, 2x/a2 (2y/b2) (dy/dx) = 0 dy/dx =



If Y X Is A Solution Of 2 Sinx 1 Y Dy Dx Cosx And Y 0 1 Then Find The Value Of Y P 2 Studyrankersonline




If Y Xx Prove That D2y Dx2 1 Y Dy Dx 2 Y X 0 Explain In Great Detail Mathematics Topperlearning Com X4m0m1ww
Int (x^2 y^2 x y^3) dx dy, x=2 to 2, y=2 to 2 Natural Language;Solve your math problems using our free math solver with stepbystep solutions Our math solver supports basic math, prealgebra, algebra, trigonometry, calculus and moreClick here👆to get an answer to your question ️ Solve the differential equation (x^2 y^2) dx 2xydy = 0



Find The General Solution Of Differential Equation X 1 Y 2 Dx Y 1 X 2 Dy 0 Sarthaks Econnect Largest Online Education Community




Solution Solve The Differential Equation X Y 1 Dx X 1 Dy 0 If Y 2 When X 1
A first order Differential Equation is Homogeneous when it can be in this form dy dx = F ( y x ) We can solve it using Separation of Variables but first we create a new variable v = y x v = y x which is also y = vx And dy dx = d (vx) dx = v dx dx x dv dx (by the Product Rule) Which can be simplified to dy dx = v x dv dxSum Rule \int f\left(x\right)\pm g\left(x\right)dx=\int f\left(x\right)dx\pm \int g\left(x\right)dx I came across a question solve $$\frac{dy}{dx} = \frac{1}{xy^2},\qquad y(2) = 0$$ I have no clue how to proceed I have suspicion that this equation has no closed form solution and may be there was a mistake in the question Course is a basic course on ODEs I will appreciate solution/hints/further reading



1




If Y X Is The Solution Of Dy Dx X 2 1 Y 2 Y 0 2 Then Y 3 Homeworklib
On substituting the value of x = 1 and y = 1, we get ⇒ dy / dx = not defined anything divided by 0 is not defined Therefore, the differentiation of the expression 3x 2 2xy y 2 = 2 at x = 1 is not defined Explore math program Explore coding program Pick your preferred day & timeSee the answer (x^2 1)dy/dx 2y = (x1)^2 put in standard form, dy/dx 2/ (x^2 1) *y = x1 /x1 how did that happen??Simple and best practice solution for (2xy)dy(x^2y^21)dx=0 equation Check how easy it is, and learn it for the future Our solution is simple, and easy to understand, so don`t hesitate to use it as a solution of your homework If it's not what You are looking for type in the equation solver your own equation and let us solve it




Worked Example Implicit Differentiation Video Khan Academy




Solve The Differential Equation X 1 Dydx 2x 3y
Let's simplify it First dy/dx = (y/x 1)/(y/x 1) Taking y = vx dy/dx = v xdv/dx Therefore, dx/x = (v 1)dv / (v^2 1) Integrating we get log (1/x) logc = arctan (y/x) 1/2 logMultiply − 2 2 by − 1 1 Since − 1 1 is constant with respect to x x, the derivative of − 1 1 with respect to x x is 0 0 Simplify the expression Tap for more steps Multiply 1 x 2 1 x 2 by 0 0 Add 2 x − 3 2 x 3 and 0 0 Rewrite the expression using the negative exponent rule b−n = 1 bn b n = 1Simple one The given differential equation can be written as (dy/dx) (y/x) = 3/x which in now in standard form of first order linear differential equation ;



1
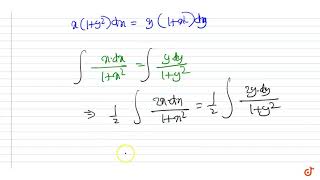



X 1 Y 2 Dx Y 1 X 2 Dy 0 Youtube
Help is appreciated Edit(1 X) (1 Y2) Dx (1 Y) (1 X2) Dy = 0 CBSE CBSE (Science) Class 12 Question Papers 1851 Textbook Solutions Important Solutions 4562 Question Bank Solutions Concept Notes & Videos 725 Time Tables 18 Syllabus Advertisement Remove all adsIf y = 2^x, find dy/dx Q If y = 2^x, find dy/dx ANSWER 1) Take Logs of both sides of our equation y = 2^x So we get log (y)=log (2^x) 2) Apply relevant log rule to rhs Log rule log (a^b) = b log (a) nb the dot between b and log (a) represents x / multiply / times ) So we get log (y) = x log (2)



Secure Media Collegeboard Org Digitalservices Pdf Ap Ap16 Calculus Ab Q4 Pdf




Find A Function Y F X Satisfying The Given Chegg Com
Dy g x h y dx Example 22dy xydx 2 2 dyx dxy 2 2 y dyx dx 2 2 y dyx dx 1 21 2 y from MATH CALCULUS at San Francisco State UniversityExtended Keyboard Examples Upload Random Compute answers using Wolfram's breakthrough technology & knowledgebase, relied on by millions of students & professionals For math, science, nutrition, history, geography, engineering, mathematics, linguistics, sports Solution Let the Given function x=\frac {23z} {z1} This is inverse of f in terms of the variable z By replacing z by x, we shall obtain inverse of f (x) in terms of the variable x c Differentiating with respect to x is the required derivative of f^1 (x)
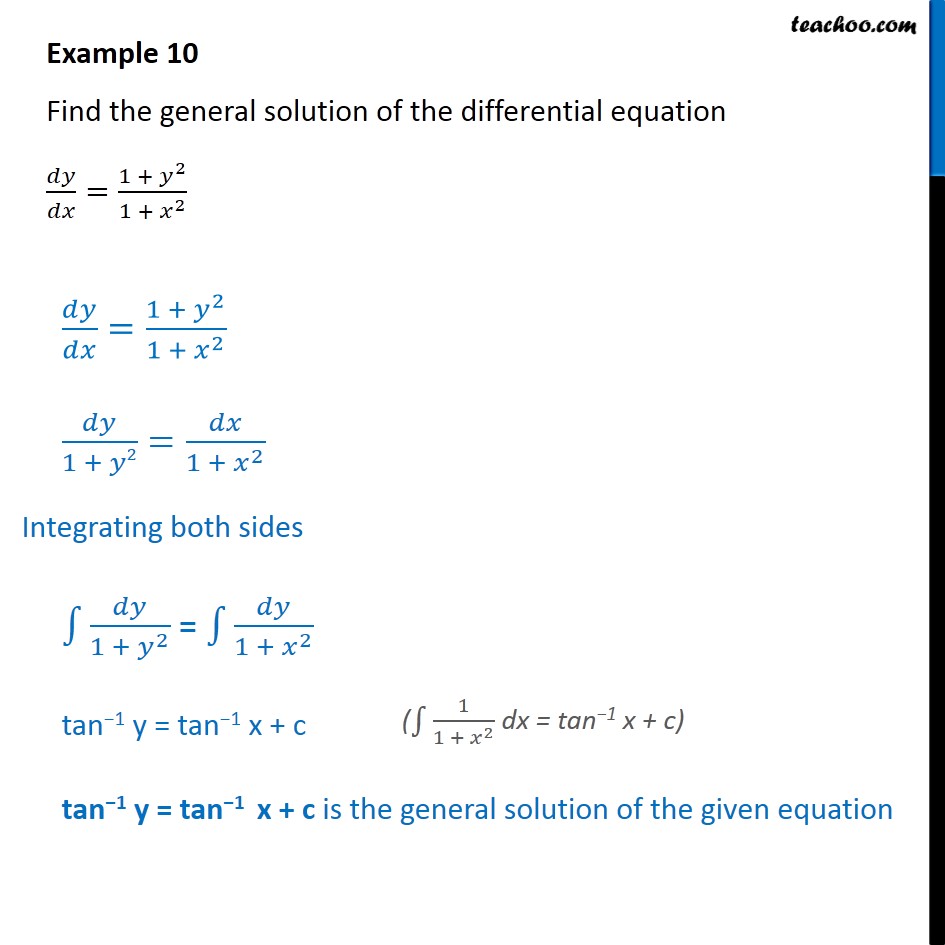



Example 10 Find General Solution Dy Dx 1 Y2 1 X2 Examples




If Y X Is The Solution Of Dy Dx X 2 1 Y 2 Y 0 2 Then Y 3 Homeworklib
Instead follow this method, Substitute y^2 = u and solve, ie keeping u as a variable find du/dx in terms of dy/dx and substitute also substitute the value of y in the expression sandeep 15 Points 2 years ago Ans dy/dx = (x^2y^21)/2xy y^2 = v rewrite the equation in the form of linear equation Then solve it (1x^2)dy/dx xy = 1/ (1x^2) the ans given is y= x/ (1x^2) C / ( sqrt rt (1x^2) ) , my ans is different , which part is wrong ?Y 1 y 1 dy = x 2 x 3 dx 3 To evaluate the integrals Z y 1 y 1 dy = Z x 2 x 3 dx we need usubstitution on both sides On the LHS, let u = y 1 and then du = dy and y = u1 On the RHS we need another variable name, so let w = x 3 and then dw = dx and x = w 3 Substituting (01




Implicit Differentiation




Differential Equations Separation Of Variables Ppt Download
Homework Solutions MATH 32B2 (18W) Problem 10 () Sketch the region Dbetween y= x2 and y= x(1 x) Express Das a simple region and calculate the integral of f(x;y) = 2yover DSolutions to x^2 dy^2 = 1 and x^2 dy^2 = 1 Recall from the Pell's Equation page that Pell's equation is $x^2 dy^2 = N$ where $d, N \in \mathbb{Z}$ We noted that Pell's equation has at most finitely many solutions if $d < 0$ or $d$ is a perfect square We will now look at a special type of Pell's equation and when it has solutions, namely (1) Solve the differential equation (x^2 1) dy/dx 2xy = 2/(x^2 1), where x ∈ ( ∞, 1) ⋃ (1, ∞) askedMar 13in Differential Equationsby Yaad(352kpoints) differential equations class12 0votes 1answer Solve the following differential equation 2x^2 dy/dx 2xy y^2 = 0



Solve Dy Dx Xy 1 X 2 X Y Sarthaks Econnect Largest Online Education Community



Secure Media Collegeboard Org Digitalservices Pdf Ap Ap16 Calculus Ab Q4 Pdf



Find The Particular Solution Of The Differential Equation X X 2 1 Dy Dx 1 Y 0 When X 2 Sarthaks Econnect Largest Online Education Community



Solved Find The Particular Solution Indicated For The Following Course Hero



What Is The Solution Of The Differential Equation 1 X 2 Dy 1 Y 2 Dx 0 Quora




If Y X X Prove That D 2y Dx 2 1 Y Dy Dx 2 Y X 0 Brainly In
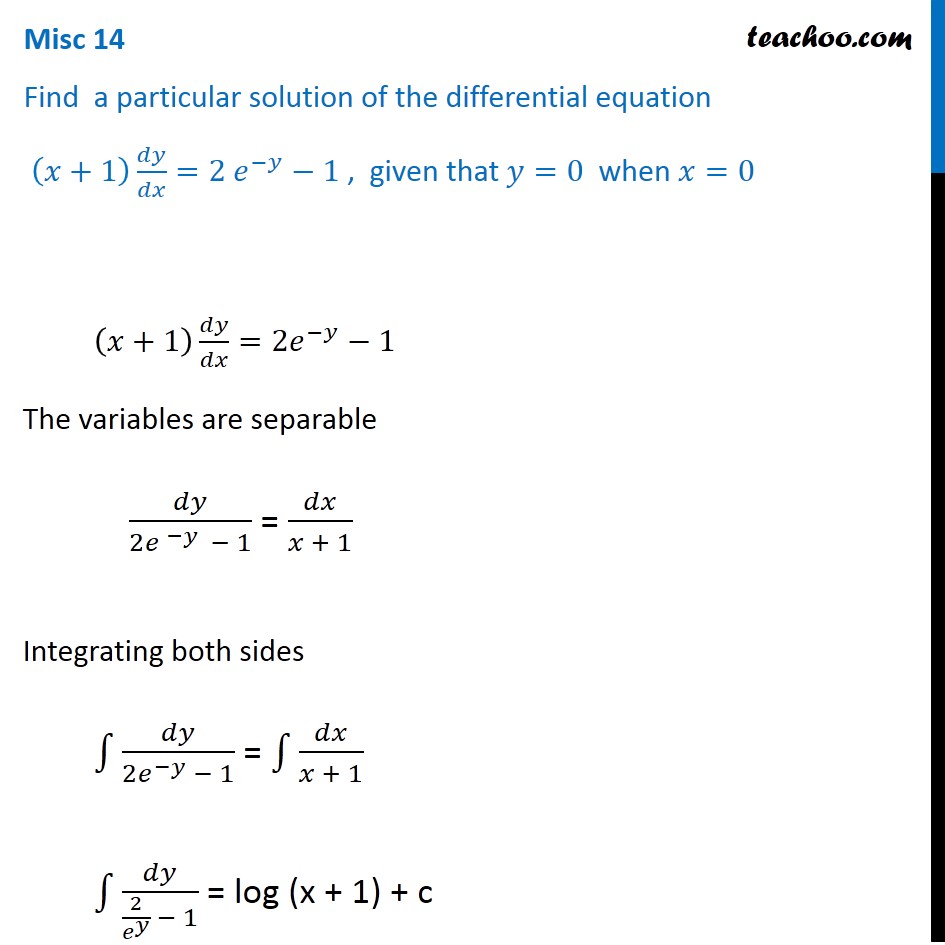



Misc 14 Find Particular Solution X 1 Dy Dx 2e Y 1




Differential Equations First Order Linear X 2 1 Dy Dx 2y X 1 2 Method Of Integrating Factor Youtube




If Y X X2 1 1 2 M Then Show That X2 1 D2y Dx2 X Dy Dx M2y 0 Mathematics Topperlearning Com 6jd9kell




Resonance Aieee Iit Study Material Maths Complete Pdf By S Dharmaraj Issuu




The Solution Of Dy Dx X 2 Y 2 2x 2 Is




Solve The Differential Equation X 1 Dy Dx 2xy Given That Y 2 1



What Is The Solution Of X 1 Y 2 Dx Y 1 X 2 Dy 0 Quora



Www3 Nd Edu Craicu 1bspring10 Quiz1b Q10 Pdf




Solve The Differential Equation Left 1 X 2 Right Frac Dy Dx 2xy 4 X 2 0 Subject To Initial Condition Yleft 0right 0 Snapsolve



Exact Differential Equation 2xy 3 Dx X 2 1 Dy 0 Youtube




Solve Y 1 Xy Dx X 1 Xy X 2y 2 Dy 0 Mathematics Stack Exchange




Math 432 Hw 2 5 Solutions Pdf Free Download




Solve The Following Differential Equation X Sqrt 1 Y 2 Dx Y Sqrt 1 X 2 Dy 0




In Solve The Given Initial Value Problem X Y 2 Chegg Com



Http Www Math Sci Hokudai Ac Jp S Settepanella Teachingfile Calculus Calculus2 Pagine Lineintex Pdf



Www Ucl Ac Uk Ucahhwi Gm01 Ode Extra Pdf




Hw 2 5 Solutions Pdf
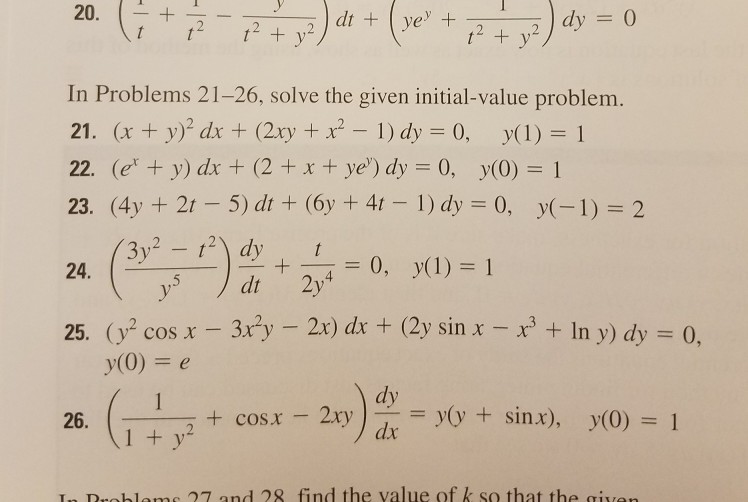



In Problems 21 26 Solve The Given Initial Value Chegg Com



1



Secure Media Collegeboard Org Digitalservices Pdf Ap Ap16 Calculus Ab Q4 Pdf



How To Find Dy Dx Of The Function Y X 1 X 2 X 1 2 Quora
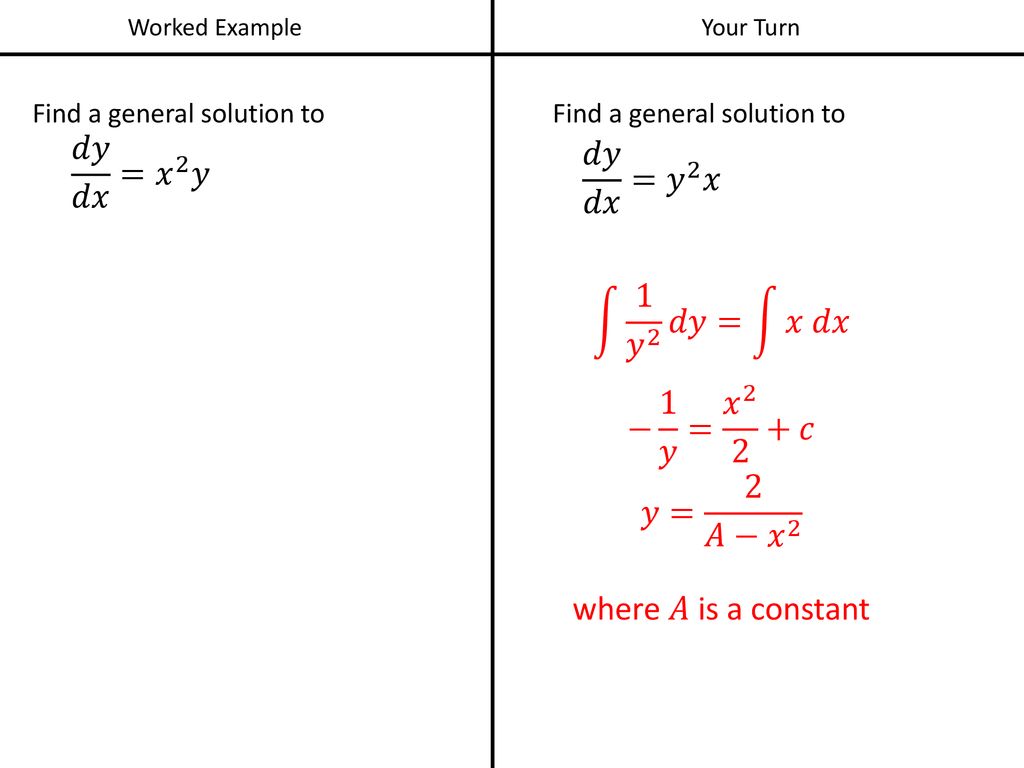



Differential Equations Separation Of Variables Ppt Download



Q Tbn And9gcqcy7j5w4xcdscte 4k9jnh7k0ovqpilkoaonby36iq6geciyka Usqp Cau




Math 432 Hw 2 5 Solutions



Vbgct8wp0ezcnm



Find The General Solution And Solve Ivp If Any Of Chegg Com




Differential Equations Separation Of Variables Ppt Download




The Solution Of The Differential Equation X Left X 2 1 Right D Y D X Y Left 1 X




Differential Equations Solved Examples Solve The Ivp 2xy X 4 Dx X 2 Y 2 Dy 0 Y 0 1



Solved Solve The Following Differential Equations X Y 3 Dx X Y 1 Dy 0 2 X Y 1 Dx 3x 4y 2 Dy C 3 1 Y 2 Xy 2 Dx X 2y Y 2xy Dy Course Hero



First Order Differential Equations Chapter 2 Ch2 2 Contents 2 1 Solution Curves Without A Solution 2 1 Solution Curves Without A Solution 2 2 Separable Ppt Download
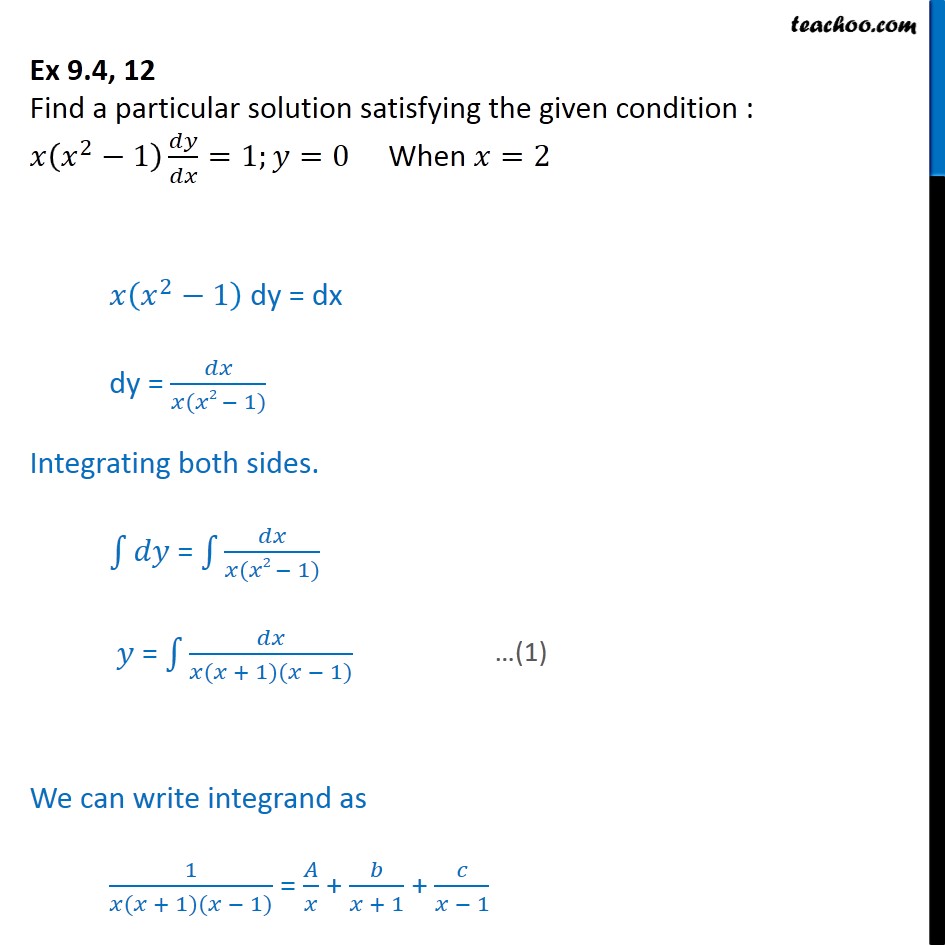



Ex 9 4 12 Find Particular Solution X X2 1 Dy Dx 1 Y 0
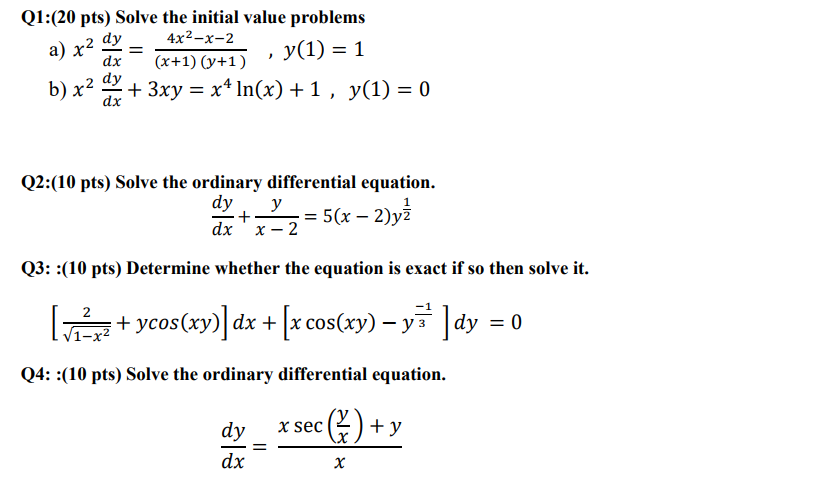



Solve The Initial Value Problems A X 2 Dy Dx 4x 2 Chegg Com




Solve The Following Differential Equation X 2 1 Dy Dx 2 X Y 2 X 2 1




X 1 Y2 Dx Y 1 X2 Dy 0 Brainly In
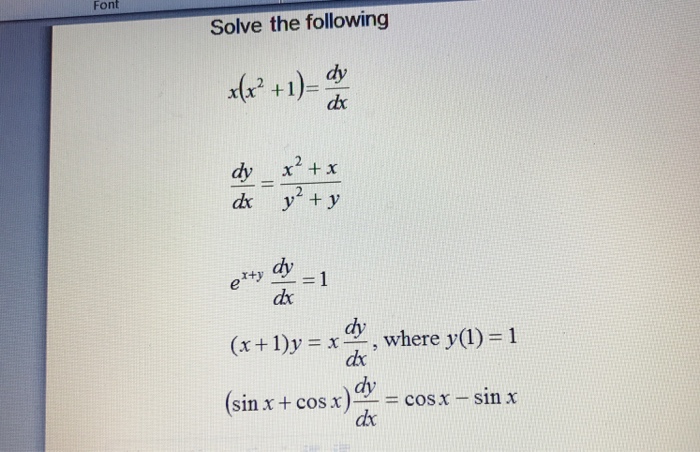



Solve The Following X X 2 1 Dy Dx Dy Dx X 2 Chegg Com
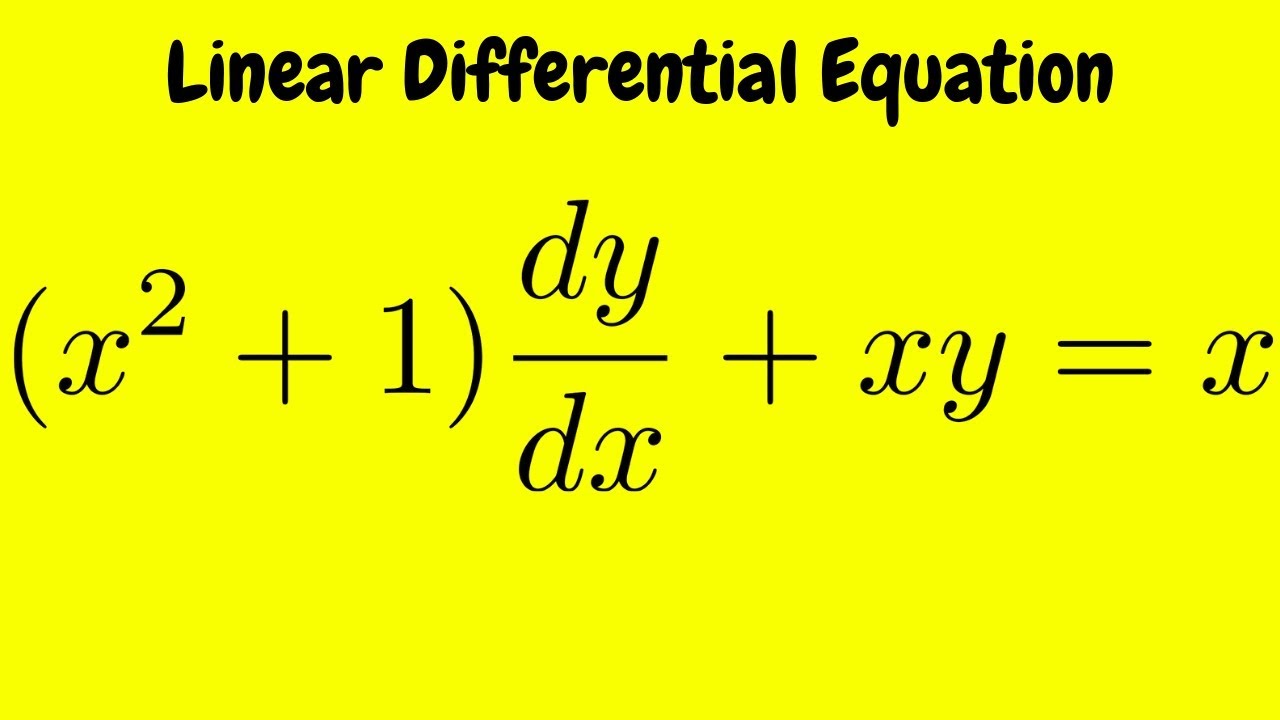



Solve The Linear Differential Equation X 2 1 Dy Dx Xy X Youtube
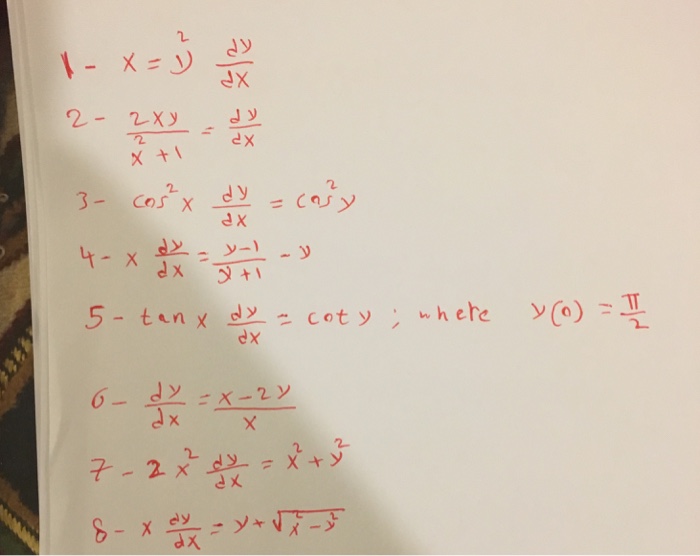



X Y 2 Dy Dx 2xy X 2 1 Dy Dx Cos 2x Dy Dx Chegg Com
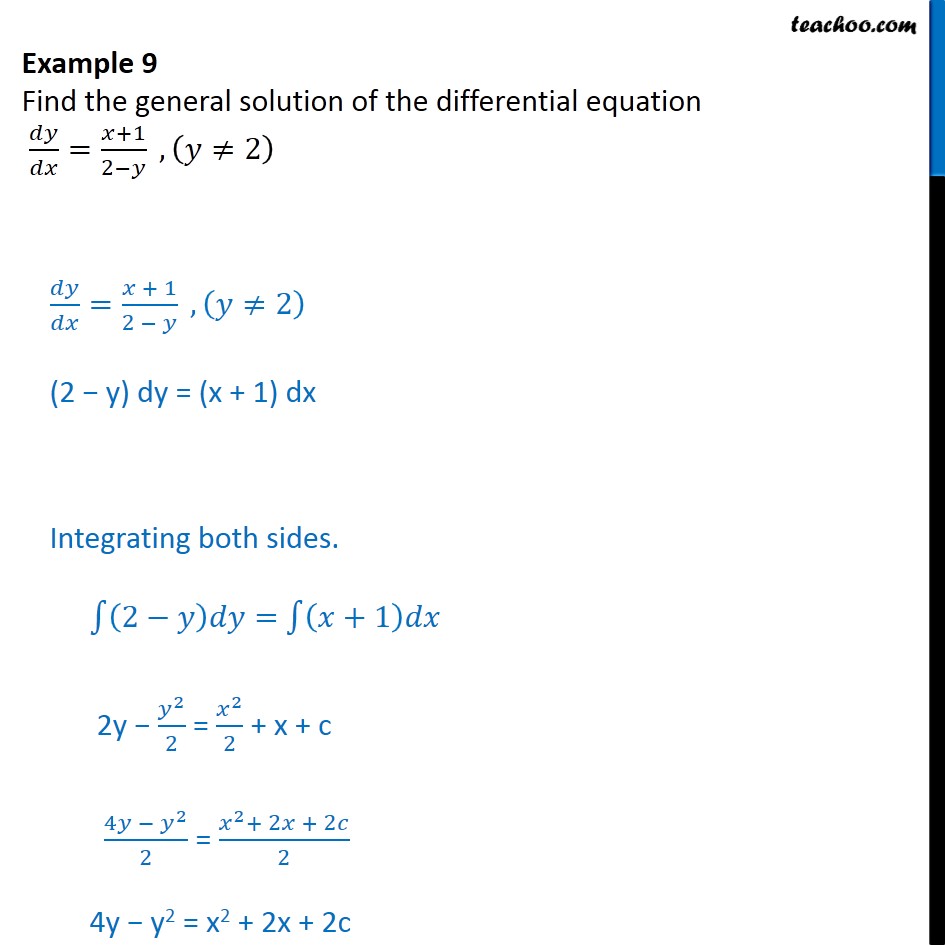



Example 9 Find General Solution Of Dy Dx X 1 2 Y Examples




Find A Solution Of The Ivp Dy Dx Xy 3 1 X 2 1 2 Y 0 1 And Give The Interval Where The Solution Is Homeworklib
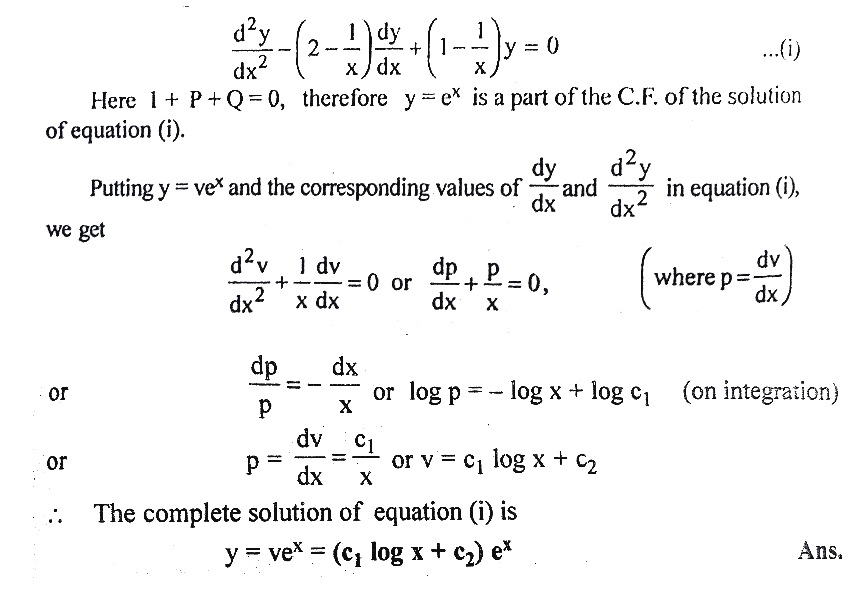



Solve X D 2 Dx 2 2x 1 Dy Dx X 1 Y 0 Given That Y E X Is An Integral Included In The Complementary Function सम करण X D 2 Dx 2 2x 1 Dy Dx X 1 Y 0 क हल क ज ए द य गय ह क Y E X




Find Dy Dx For The Given Function Y X 1 X 2 X Brainly In




Solve X X 1 Dy Dx X 2 Y X3 2x 1 Maths Application Of Derivatives Meritnation Com




Worked Example Identifying Separable Equations Video Khan Academy



Www Ualberta Ca Csproat Homework Math 215 Solution 3 Pdf



Secure Media Collegeboard Org Digitalservices Pdf Ap Ap16 Calculus Ab Q4 Pdf



How To Solve This Differential Equation X 1 Dy Dx X 2 3x 2 Quora
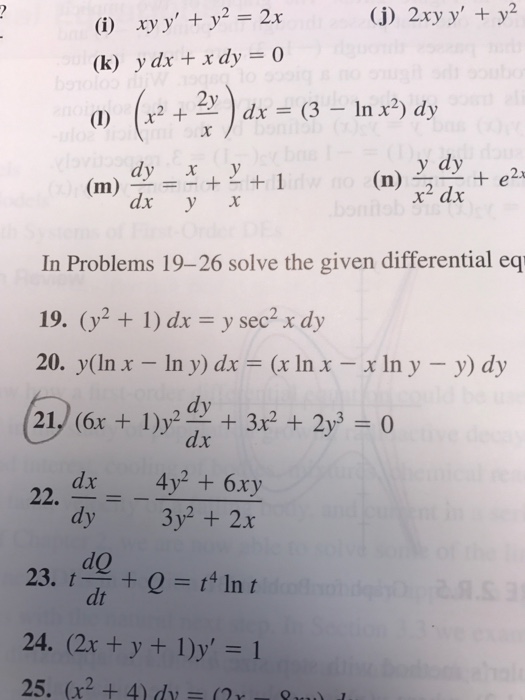



Xy Y Y 2 2x 2xy Y Y 2 Y Dx X Dy 0 X 2 Chegg Com
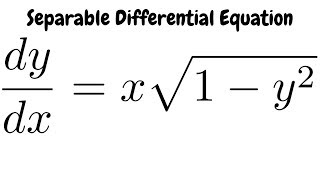



Separable Differential Equation Dy Dx X Sqrt 1 Y 2 Youtube




The Solution Of Dy Dx X 2 Y 2 1 2xy Satisfying Y 1 0 Is Given By
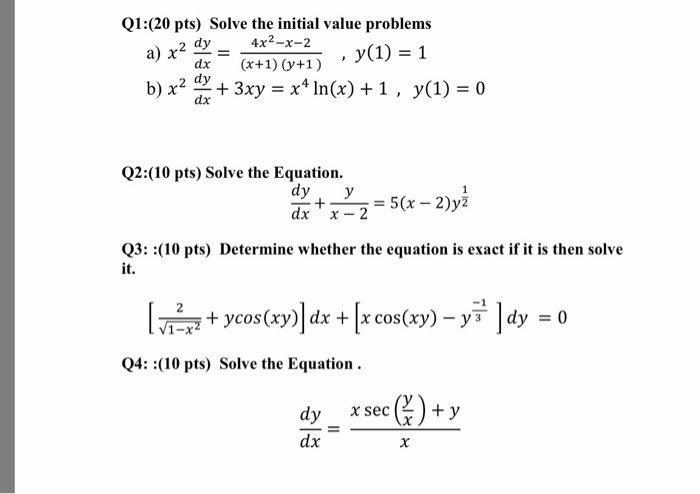



Solve The Initial Value Problems X 2 Dy Dx 4x 2 Chegg Com
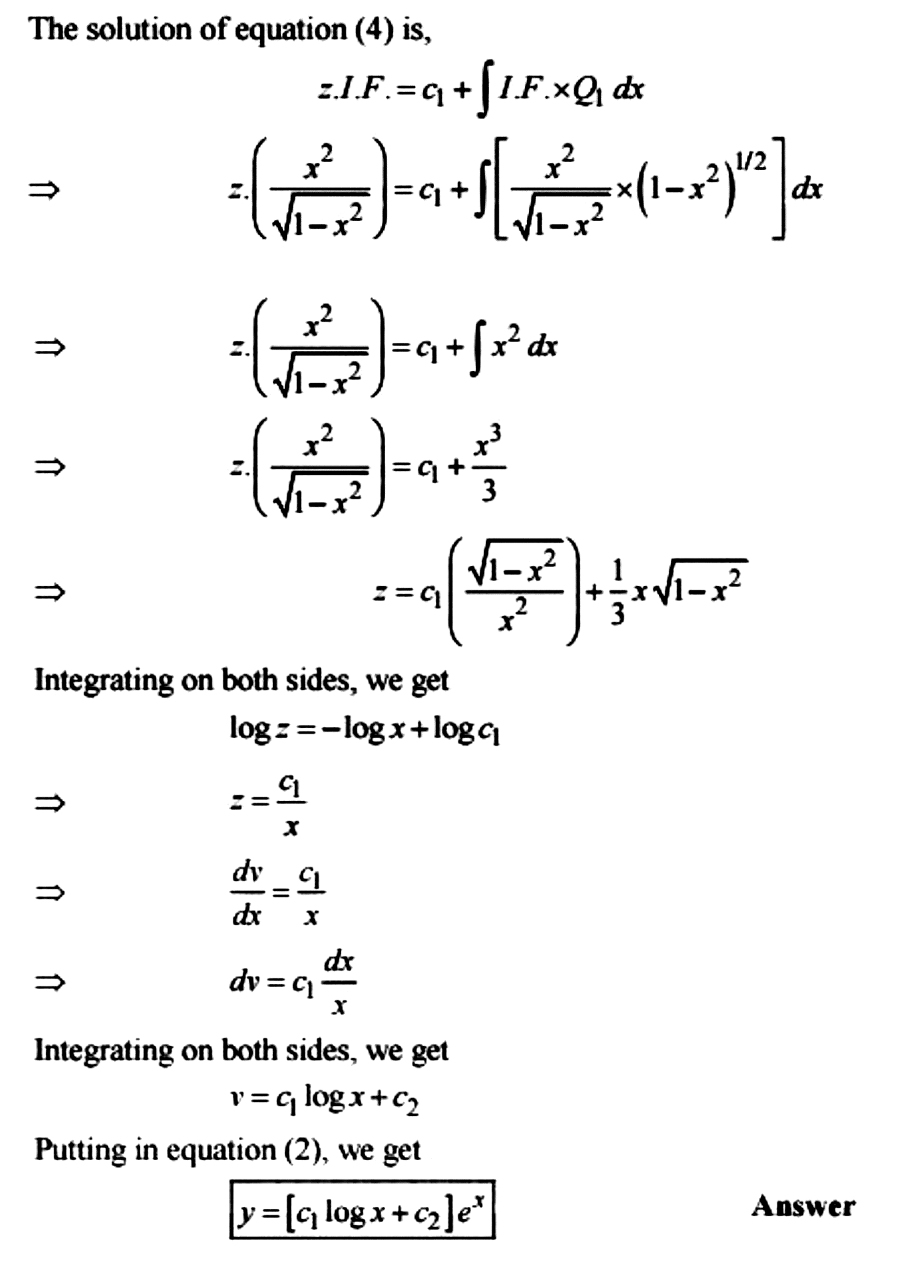



Solve 1 X 2 D 2y Dx 2 X Dy Dx Y X 1 X 2 3 2 Mathematics 2 Question Answer Collection




Homogeneous Differential Equations
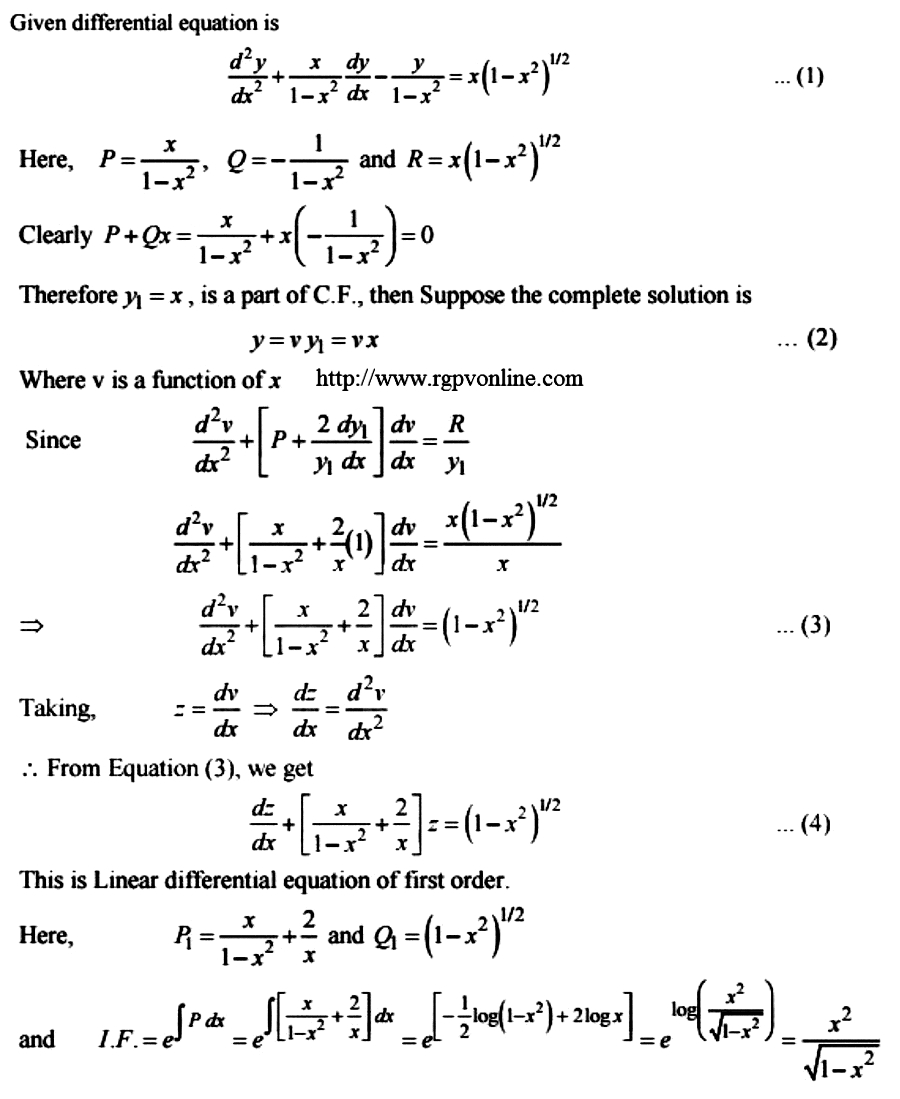



Solve 1 X 2 D 2y Dx 2 X Dy Dx Y X 1 X 2 3 2 Mathematics 2 Question Answer Collection



Solve The Differential Equation X2 1 Dy Dx 2xy 1 X2 1 Studyrankersonline




For Problems 26 Determine An Integrating Factor Chegg Com



Answers To The Review Problems For The First Exam 251 05 10 In Spring 06




The Solution Of The Differential Equation X Left X 2 1 Right D Y D X Y Left 1 X



What Is The Solution To This Differential Equation X 2 Y 2 1 Dx X X 2y Dy 0 Quora



1 X 2 Dy Dx Xy 1 Youtube



Find The General Solution Of Differential Equation X 2 Y 1 Dx Y 2 X 1 Dy 0 Sarthaks Econnect Largest Online Education Community




Prove That Frac Dy Dx Frac1 1 X 2 For Given That X Sqrt 1 Y Y Sqrt 1 X 0 Mathematics Stack Exchange



Qkpba2ytmixmim




Practice Questions Calculus Xii
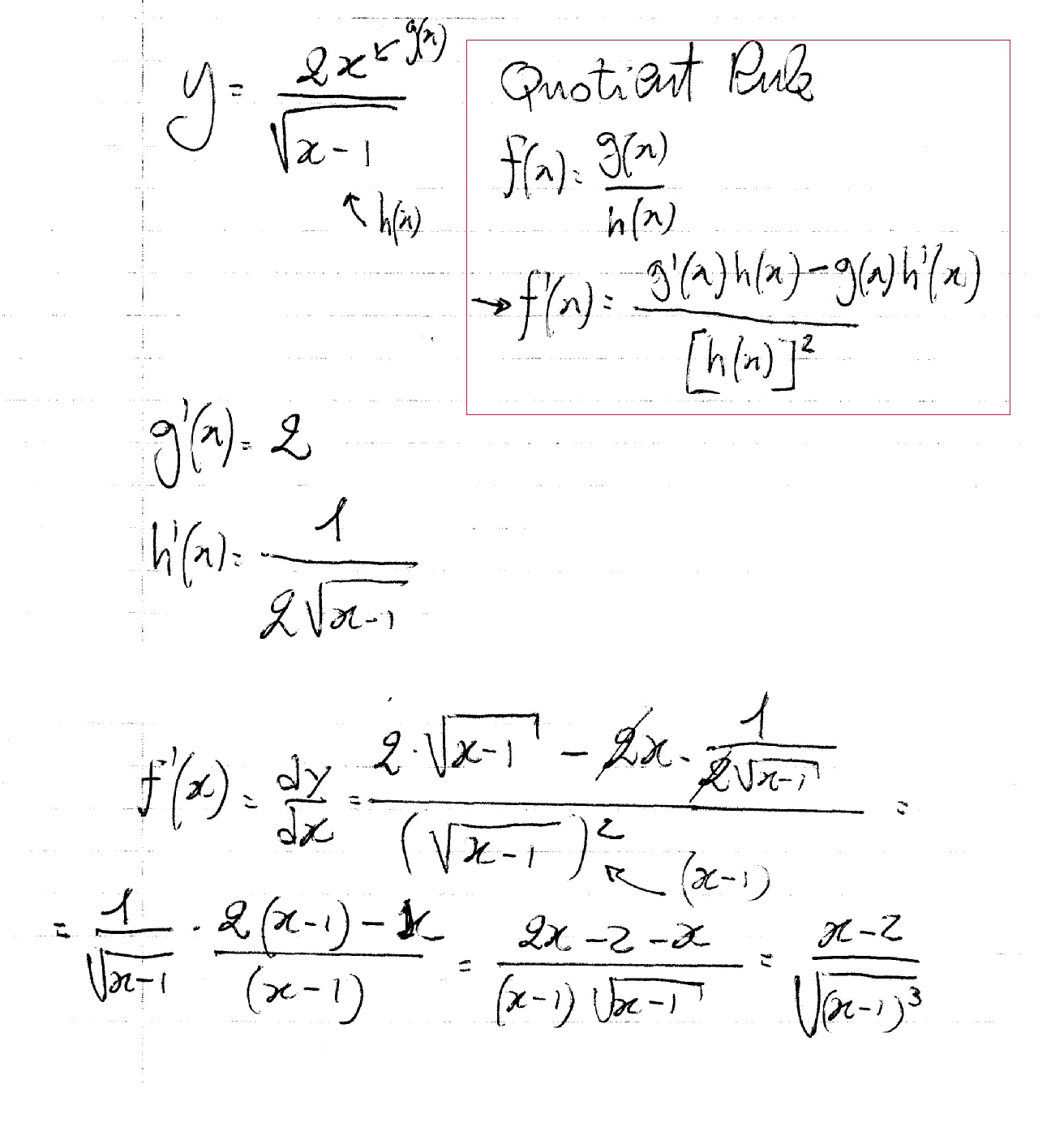



How Do You Find Dy Dx For Y 2x Sqrt X 1 Socratic




Engineering Mathematics Notes




Solve X 2 Yx 2 Dy Y 2 Xy 2 Dx 0




Solve X X 1 Dy Dx Y X2 X 1 2 Maths Differential Equations Meritnation Com



Http 1 160 97 198 8080 Xmlui Bitstream Handle 2 2 chapter 1 11 Pdf Sequence 2



Bernoulli Differential Equation Dy Dx Y Xy 2 1 Youtube



0 件のコメント:
コメントを投稿